Geometric Mean Calculator

Need to quickly calculate the geometric mean? Our calculator will help you! Enter your data, and we will calculate the result for you. There's no need to waste time on manual calculations - the geometric mean calculator is easy to use and will quickly give you a result that is accurate to the number of decimal places you choose.

About the Geometric Mean Calculator

The geometric mean calculator is easy to use and allows you to quickly calculate the mean of the product of several numbers. To use the calculator, all you have to do is enter your data in the text box, separating them with semicolons. You can also choose how precise you want the result to be by specifying the number of decimal places. Any change in the text box or precision will automatically recalculate and display the result. Use our calculator today and quickly calculate the geometric mean.

What is the geometric mean?

The geometric mean is a mathematical indicator of the average that is used when you want to calculate the arithmetic mean of multiplying several numbers. In other words, the geometric mean is the square root to the nth power of the product of n numbers. It is often used when you want to find the arithmetic mean of data that are very different or linearly correlated. It can also be used in many fields such as finance, statistics, life sciences, and engineering. Note, however, that the geometric mean is not well suited for data that are not linearly correlated and cannot be calculated for negative values.

Definition: The geometric mean of two or more numbers x1, x2, ..., xn is equal to the nth root of the product of these numbers: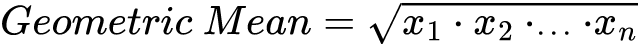

An example

To calculate the geometric mean of several numbers, simply multiply them and then find the square root of the resulting product, which is of degree n.

For example, to calculate the geometric mean of the numbers 4, 9, and 16, do the following

• multiply all the numbers together: 4 * 9 * 16 = 576
• find the square root of the product: √576 = 24

In this case, the geometric mean is 24.

Applications of the geometric mean in various fields

The geometric mean is widely used in many fields, including finance, statistics, life sciences, and engineering.

Finance: The geometric mean is often used in finance to calculate the rate of return on investments. For example, if we have data on the return on 3 different investments over the past 3 years, we can calculate the geometric mean to find the average annual return on those investments.

Statistics: The geometric mean is often used in statistics to calculate the arithmetic mean for data that are linearly correlated. For example, if we have population growth data for different countries, we can calculate the geometric mean to find the average population growth for those countries.

Life sciences: The geometric mean is often used in the life sciences to calculate the average of many physical quantities, such as chemical concentrations or body mass. For example, if we have data on the concentration of different chemicals in different samples, we can calculate the geometric mean to get the average concentration for those samples.

Technology: Geometric mean is often used in technology to calculate an average for many parameters, such as device power or screen brightness. For example, if we have power data for different devices, we can calculate the geometric mean to get the average power for those devices.

The geometric mean is widely used in many fields such as finance, statistics, life sciences, and engineering. It can be used to calculate the arithmetic mean for data that are very different or linearly correlated. It is a valuable tool to consider when analyzing data, although its limitations, such as sensitivity to negative values and computational difficulty, should be kept in mind.

Advantages and disadvantages of the use of the geometric mean

The geometric mean is a valuable mathematical tool used in many fields. However, it has several limitations that must be considered when using it.

Advantages:

• the geometric mean reflects the true distribution of the data better than the arithmetic mean when the data are very different;
• the geometric mean is immune to outliers, meaning that one or more outliers will not significantly affect the final result;
• the geometric mean is often used in statistics to calculate the average for data that are linearly correlated;

Disadvantages:

• the geometric mean is sensitive to negative values. Unlike the arithmetic mean, the geometric mean cannot be calculated for negative values;
• the geometric mean is more difficult to calculate than the arithmetic mean because it requires raising all numbers to the power of n and calculating the nth degree root;
• the geometric mean is not well suited for data that are not linearly correlated. In this case, the harmonic mean or the quadratic mean would be a better choice;

The geometric mean is a valuable mathematical tool that has many advantages, such as better representation of the true distribution of the data and robustness to outliers. It is also often used in statistics to calculate the mean for data that are linearly correlated. However, one should be aware of its limitations, such as sensitivity to negative values and computational difficulties. For data that are not linearly correlated, other measures of mean, such as harmonic mean or quadratic mean, may be a better choice.

see also: