Root Calculator (nth root)
The root calculator is a simple online tool that allows you to quickly and easily calculate the root of any number. Our calculator allows you to find the root of numbers quickly and easily. To perform a root, enter the degree of the root and the number below the root in the box below. The result will automatically appear after the equals sign. If the result is a number with decimal expansion, you can specify the precision with which it should appear, i.e. how many decimal places it should appear in the result.
How do I use the root calculator?
The root calculator allows you to calculate root of any degree. You can calculate square root (2nd degree root), cube root (3rd degree root), etc. Our root calculator is ideal for students, teachers and people working in fields where root are common. It's fast, easy to use and available online, so you can use it anytime, anywhere.
So how do you use this root calculator? First you need to enter the degree of the root (e.g. 2, 3, 4, 5, etc.) and the number you are rooting. You can also set the precision (how many digits after the decimal point) with which the result is displayed. The default is two digits after the decimal point.
As you can see, this root calculator is a very easy-to-use online tool that you can use whenever you need to calculate the root of a number of any degree.
Prime - Definition
The root of the nth degree of a number a is equal to b if b to the nth power is equal to apropriation
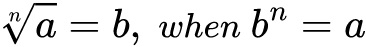
A prime is a mathematical operation that is the inverse of a power. Elements are nothing more than the inverse of powers.
When we are dealing with a root of the second degree (root of 2, n = 2), this digit is omitted from the notation. Such a root can be read as a square root.
If we are dealing with a third degree root (the root of 3, n = 3), such a root can be read as a cube root.
The square root of 3
The square root of 3 is a non-measurable number and its approximate value is: 1.73205080757. The longer expansion of this number is: 1.73205080756887729352744634150587236694280525381038062805580697945193...
The square root of 3 is also called Theodore's constant (Theodore of Cyrene - Greek mathematician, born 460 BC). Plato credited him in his text with the first proof of the indistinguishability of square root, including the number 3.
The square root of 3 is a value that appears as some dimensions in geometric figures. For example:
- an equilateral triangle with a side of 2 has a height equal to the square root of 3,
- the length of the diagonal of a cube with a side of 1 is also the square root of 3.
Summary
Elements appear in many areas of mathematics, such as algebra, equations and inequalities, geometry and mathematical analysis. They are also widely used in physics and other sciences.
Primes can be divided into square, cubic and general primes. Square primes are second-degree primes, that is, primes which, when squared, give a given number. Cubic primes are third-degree primes, i.e. primes which, when cubed, give a given number. General primes are primes of any degree.
There are formulae for calculating square and cube primes. For square root there is the Bhaskaraye formula, and for cubic root there is the Cardano formula. For general primes, the calculations can be more complicated and require more advanced mathematical methods.
Primes are very useful in many areas of mathematics, including algebra, geometry and analysis. Square primes are often used to calculate the length of the opposite side of a right triangle, and third-degree primes are often used to calculate the volume of a cube or other geometric body. Their knowledge is essential for solving many equations and inequalities, and for calculating many quantities in physics and other fields.
The square root calculator is sure to come in handy for students. It is trivial to use and the result of the operation appears instantly. The inverse of rooting is power, so if you need the right tool for this activity, take a look at our Power Calculator (#soon).
see also: