Log Calculator | Free Online Logarithm Tool
Logarithm Calculator
Quick Select Base:
Enter a positive number and select a base to calculate its logarithm.
Our Logarithm Calculator is a powerful and user-friendly online tool designed to compute logarithms quickly and accurately. Whether you're solving a math problem, analyzing scientific data, or exploring exponential relationships, this calculator simplifies the process. Simply input the number you want to find the logarithm of and specify the base—such as 2, 10, or e for the natural logarithm (ln)—and the result appears instantly.
This tool supports a variety of bases to suit your needs: use base 10 for common decimal logarithms, base 2 for binary applications (like computer science), or base e (approximately 2.71828) for natural logarithms used in calculus and physics. You can also fine-tune the precision of your result, selecting anywhere from 0 to 10 decimal places, ensuring the output matches the level of detail you require.
Quick Logarithm Reference
Common Log (base 10)
Written as log₁₀(x) or simply log(x)
Example: log₁₀(100) = 2
Natural Log (base e)
Written as loge(x) or ln(x)
Example: ln(e) = 1
Binary Log (base 2)
Written as log₂(x)
Example: log₂(8) = 3
How to Use the Log Calculator
Our Logarithm Calculator is intuitive and flexible. To get started:
- Enter the number you want to find the logarithm of (e.g., 100, 2.5, etc.)
- Select the base of the logarithm (10, e, 2, or enter a custom base)
- Choose your desired precision (decimal places)
- The result will automatically update
For example, if you set the base to 10 and the number to 100, the calculator returns log10 100 = 2, because 10 raised to the power of 2 equals 100. For the natural logarithm, enter e as the base and a number like 2.71828 (approximately e), and you'll get ln 2.71828 ≈ 1.
What is a Logarithm?
A logarithm is a mathematical operation that reveals the exponent needed to produce a given number from a specific base. In simpler terms, it answers: "To what power must I raise a to get b?" For positive numbers a and b (where a ≠ 1), the logarithm base a of b is the value x that satisfies the equation ax = b.
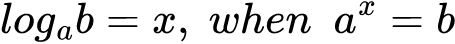
Logarithms are the inverse of exponentiation, making them crucial for "undoing" exponential growth or decay. They compress large ranges of values into manageable scales, a property that's key in fields like science, engineering, and economics.
Types of Logarithms
Decimal Logarithm (Base 10)
The decimal logarithm, also known as the common logarithm, uses a base of 10. It's often written as log x or log10 x. For example, log 1000 = 3 because 103 = 1000. This type is widely used in scientific notation and many calculations.
Natural Logarithm (Base e)
The natural logarithm has a base of e (approximately 2.718). It's denoted as ln x or loge x. For instance, ln e = 1 because e1 = e. Natural logarithms are essential in calculus, physics, and fields involving growth or decay.
Binary Logarithm (Base 2)
The binary logarithm uses a base of 2, written as log2 x. For example, log2 8 = 3 because 23 = 8. Binary logarithms are particularly important in computer science, information theory, and music.
Real-World Applications of Logarithms
Science & Engineering
- pH Scale: Measures acidity using logarithms (pH = -log[H+])
- Richter Scale: Measures earthquake intensity logarithmically
- Decibels: Sound levels follow a logarithmic scale
- Stellar Magnitude: Brightness of celestial objects
Mathematics & Computing
- Algorithm Analysis: Measuring computational efficiency
- Information Theory: Quantifying information content
- Number Theory: Solving complex equations
- Data Compression: Reducing file sizes efficiently
Logarithm Rules and Properties
| Property | Formula | Example |
|---|---|---|
| Product Rule | loga(x·y) = loga(x) + loga(y) | log(10·100) = log(10) + log(100) = 1 + 2 = 3 |
| Quotient Rule | loga(x/y) = loga(x) - loga(y) | log(1000/10) = log(1000) - log(10) = 3 - 1 = 2 |
| Power Rule | loga(xn) = n·loga(x) | log(103) = 3·log(10) = 3·1 = 3 |
| Change of Base | loga(x) = logb(x) / logb(a) | log2(8) = log(8) / log(2) ≈ 0.9031 / 0.301 = 3 |
FAQs About Logarithms
Can I calculate logarithms of negative numbers?
No, logarithms of negative numbers or zero are undefined in the real number system. The input must be positive (greater than 0).
What happens if I use 1 as the logarithm base?
A base of 1 yields undefined results because 1 raised to any power is always 1, making it impossible to solve the equation 1x = b for any b other than 1.
What's the difference between log and ln?
"log" typically refers to base-10 logarithm, while "ln" (natural logarithm) refers to base-e logarithm, where e ≈ 2.718.
How accurate is this calculator?
This calculator provides results with up to 10 decimal places of precision, sufficient for most scientific and educational applications.
Why Use Our Logarithm Calculator?
- Multiple Bases: Calculate logarithms with base 10, e (natural), 2, or any custom base
- Adjustable Precision: Choose from 0 to 10 decimal places
- Instant Results: Get answers in real-time as you type
- User-Friendly: Simple interface with clear instructions
- Free To Use: No account or subscription required
- Mobile-Friendly: Works well on all devices
see also: